I recently bought a new television capable of passive 3d. When I was trying to select the "best" television I found out there wasn't a proper easy way to measure 3d crosstalk that is the most important feature of 3d technology. It's equally important as stereo crosstalk in audio signals but nowadays most audio signals are so high fidelity that audio crosstalk is basically non-existent.
But 3d technology still has to evolve, still too much crosstalk and to push the technology forward the consumers have to know which technology to buy and which not. One way of doing that is figuring how to measure 3d crosstalk preferable without any meters but visually and doing it so that results are interchangeable between different televisions settings and 3d technologies.
One of important settings will be gamma curve. Some televisions have gamma curve 2.2, some 2.4, others something else. The gamma curve defines how the television shows signals color levels as luminance levels in screen. If gamma curve is 1.0, zero signal shows zero light, 1 signal shows full light and 1/2 signal shows half light. But normal gamma curves used (2.2, 2.3, 2.4 ) means that 1/2 signal is not shown as half light but less than that.
If you don't know what gamma curve your television is set to, it probably is 2.2.
With 3d glasses, left eye sees only picture intended for left eye and vice versa. But technology is not perfect and left eye sees also a little bit of picture intended for the right eye. That is 3d crosstalk and in movies it shows as ghost images.
Static contrast ratio is the ratio between the whitest white and blackest black in static picture. If you have OLED television, then it's contrast ratio is virtually infinite. Others have to live with little imperfection and for instance my televisions static contrast ratio should be about 1:3000. The 3d crosstalk can also be presented as a ratio between the "right" picture and the "wrong" picture.
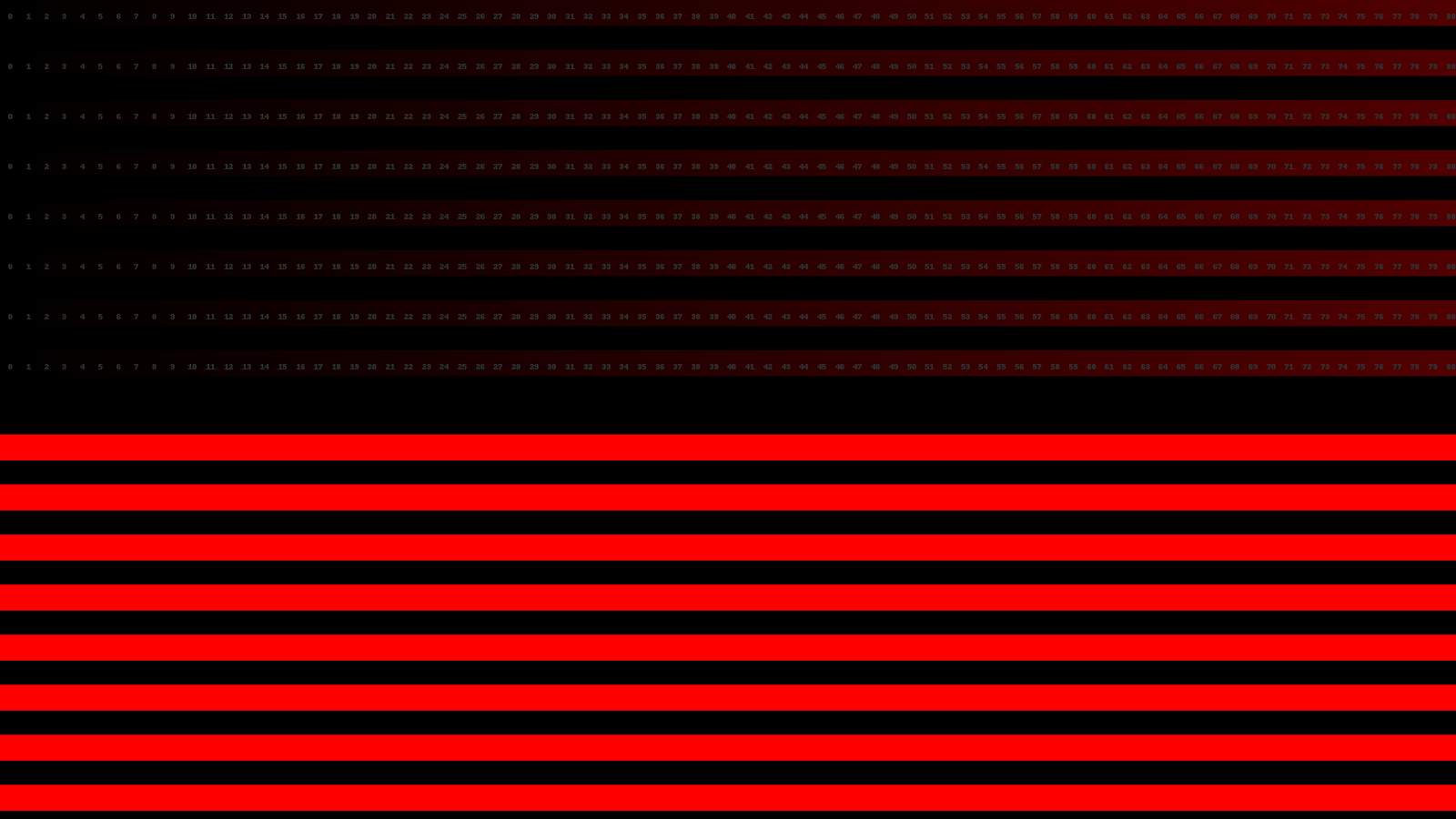
The test charts have the "right" picture that is different patches starting from black patch and moving to patches with more luminance. The "wrong" picture is full white stripes. When you are testing with left-eye-pictures, you put a piece of paper or your hand in front of your right eye to block it and and only your left eye sees the picture through the 3d glasses. Then you see the "right" picture as you should and the "wrong" picture as faint but you can still see the with stripes.
Now you have to select which of the "right" pictures patches equals the "wrong" pictures faint white stripe in luminosity. The faint white stripe is the crosstalk and you are trying to measure it. If it is too difficult to find the correct patch, you can also try to find the first and last patch that look similar as the white patch. Then take the luminosity values from the table below and the right patch is the one that has luminosity (first patch luminosity + last patch luminosity )/2 eg. the average of the two.
Luminosity for patches:
When you are viewing picture for your left eye, the patch stripe consists of two pictures, the left eye patch and the black portion of the right side picture.The black is not completely black and it depends of televisions contrast ratio. In calculations we normalize the luminance that complete black is 0 and full white is 1. There is no complete black ( unless you have OLED) so in our calculations black is the contrast ratio CR. If my tv has CR 1:3000 then luminance of black will be 1/3000.
We'll call 3d crosstalk contrast as 3dCR.
The patch luminance of "right" picture will be ( CR + power(patch,gamma) *(1-CR)) because the patch number has to be transformed to linear values through gamma power function and then it has to fit between values CR and 1. The luminance of "wrong" picture will be black multilpied by 3dCR.
Then the luminance of the patch you selected is:
( CR + power(patch,gamma) *(1-CR)) + CR*3dCR
The white stripe ( the "wrong" picture ) consists of black of "right" picture and white of "wrong" picture multiplied by 3dCR.
Then the white stripe luminance is: CR + 1 * 3dCR
Because you selected the patch that equals the white stripe:
( CR + power(patch,gamma) *(1-CR)) + CR*3dCR = CR + 1 * 3dCR
I'll then solve 3dCR:
( CR + power(patch,gamma) *(1-CR)) + CR*3dCR = CR + 1 * 3dCR
CR + power(patch,gamma) *(1-CR) + CR*3dCR = CR + 1 * 3dCR
power(patch,gamma) *(1-CR) + CR*3dCR = 1 * 3dCR
power(patch,gamma) *(1-CR) = 1 * 3dCR - CR*3dCR
power(patch,gamma) *(1-CR) = 3dCR * (1 - CR)
power(patch,gamma) = 3dCR
The equation seemed difficult at first but in the end only the patch luminance is needed for 3d contrast ratio!
I've precalculated 3d contrast ratio ( = 1/3dCR) values for different gamma values:
So if I have selected patch number 37 for blue color and my gamma is 2.4, the 3d contrast ratio for blue is 1:103 which means less that 1% of blue crosstalk. The table also shows why knowing your gamma setting is important.
You can calculate your white 3d contrast with this formula:
The white contrast = (
( Red contrast * 0,212655 ) +
( Green contrast * 0,715158 ) +
( Blue contrast * 0,072187 )
)
The test pictures with 60 patches:
And the white ones just for curiosity:
And the additional test pictures with 80 patches:
But 3d technology still has to evolve, still too much crosstalk and to push the technology forward the consumers have to know which technology to buy and which not. One way of doing that is figuring how to measure 3d crosstalk preferable without any meters but visually and doing it so that results are interchangeable between different televisions settings and 3d technologies.
One of important settings will be gamma curve. Some televisions have gamma curve 2.2, some 2.4, others something else. The gamma curve defines how the television shows signals color levels as luminance levels in screen. If gamma curve is 1.0, zero signal shows zero light, 1 signal shows full light and 1/2 signal shows half light. But normal gamma curves used (2.2, 2.3, 2.4 ) means that 1/2 signal is not shown as half light but less than that.
If you don't know what gamma curve your television is set to, it probably is 2.2.
With 3d glasses, left eye sees only picture intended for left eye and vice versa. But technology is not perfect and left eye sees also a little bit of picture intended for the right eye. That is 3d crosstalk and in movies it shows as ghost images.
Static contrast ratio is the ratio between the whitest white and blackest black in static picture. If you have OLED television, then it's contrast ratio is virtually infinite. Others have to live with little imperfection and for instance my televisions static contrast ratio should be about 1:3000. The 3d crosstalk can also be presented as a ratio between the "right" picture and the "wrong" picture.
How test patterns work
In my test patterns I have divided three main colors to separate test charts and both eyes have their own test charts so there are six different test charts. There is also test charts for white color but problem with white test chart is that your television might have for instance more crosstalk for instance blue color so you would have to try to match blue color with white color and it's difficult to do without a meter. It it easier to measure each color separately.
The test charts have the "right" picture that is different patches starting from black patch and moving to patches with more luminance. The "wrong" picture is full white stripes. When you are testing with left-eye-pictures, you put a piece of paper or your hand in front of your right eye to block it and and only your left eye sees the picture through the 3d glasses. Then you see the "right" picture as you should and the "wrong" picture as faint but you can still see the with stripes.
Selecting the right patch
Now you have to select which of the "right" pictures patches equals the "wrong" pictures faint white stripe in luminosity. The faint white stripe is the crosstalk and you are trying to measure it. If it is too difficult to find the correct patch, you can also try to find the first and last patch that look similar as the white patch. Then take the luminosity values from the table below and the right patch is the one that has luminosity (first patch luminosity + last patch luminosity )/2 eg. the average of the two.
Luminosity for patches:
| Patch | 2,2 | 2,3 | 2,4 |
| 0 | 0 | 0 | 0 |
| 1 | 5,07705190066176E-006 | 2,91714209990719E-006 | 1,67611405153091E-006 |
| 2 | 0,000023328 | 1,4365692796936E-005 | 8,84658300141058E-006 |
| 3 | 5,69217657121931E-005 | 3,65036650558521E-005 | 2,34096315502103E-005 |
| 4 | 0,0001071874 | 0,000070745 | 4,66925450146811E-005 |
| 5 | 0,000175124 | 0,0001181921 | 7,97685278983462E-005 |
| 6 | 0,0002615438 | 0,0001797651 | 0,0001235568 |
| 7 | 0,0003671363 | 0,0002562613 | 0,0001788706 |
| 8 | 0,0004925038 | 0,0003483891 | 0,0002464447 |
| 9 | 0,0006381828 | 0,0004567887 | 0,0003269532 |
| 10 | 0,0008046585 | 0,0005820464 | 0,0004210208 |
| 11 | 0,0009923743 | 0,0007247042 | 0,0005292319 |
| 12 | 0,0012017395 | 0,0008852674 | 0,0006521366 |
| 13 | 0,0014331346 | 0,0010642099 | 0,0007902557 |
| 14 | 0,0016869153 | 0,0012619788 | 0,0009440845 |
| 15 | 0,0019634162 | 0,0014789976 | 0,0011140959 |
| 16 | 0,0022629532 | 0,0017156692 | 0,0013007431 |
| 17 | 0,0025858256 | 0,0019723778 | 0,0015044612 |
| 18 | 0,0029323183 | 0,0022494914 | 0,0017256693 |
| 19 | 0,003302703 | 0,0025473632 | 0,0019647722 |
| 20 | 0,0036972396 | 0,0028663325 | 0,0022221612 |
| 21 | 0,0041161771 | 0,0032067268 | 0,0024982154 |
| 22 | 0,0045597549 | 0,003568862 | 0,0027933027 |
| 23 | 0,0050282035 | 0,0039530436 | 0,0031077808 |
| 24 | 0,0055217449 | 0,0043595678 | 0,0034419975 |
| 25 | 0,0060405937 | 0,0047887218 | 0,0037962918 |
| 26 | 0,0065849574 | 0,0052407843 | 0,0041709944 |
| 27 | 0,007155037 | 0,0057160266 | 0,0045664279 |
| 28 | 0,0077510274 | 0,0062147127 | 0,0049829077 |
| 29 | 0,0083731177 | 0,0067370998 | 0,0054207423 |
| 30 | 0,0090214919 | 0,0072834387 | 0,0058802336 |
| 31 | 0,0096963287 | 0,0078539744 | 0,0063616773 |
| 32 | 0,0103978023 | 0,008448946 | 0,0068653632 |
| 33 | 0,0111260824 | 0,0090685875 | 0,0073915756 |
| 34 | 0,0118813344 | 0,0097131276 | 0,0079405936 |
| 35 | 0,01266372 | 0,0103827904 | 0,0085126911 |
| 36 | 0,0134733969 | 0,0110777953 | 0,0091081373 |
| 37 | 0,0143105194 | 0,0117983575 | 0,0097271968 |
| 38 | 0,0151752382 | 0,0125446878 | 0,01037013 |
| 39 | 0,0160677009 | 0,0133169933 | 0,0110371927 |
| 40 | 0,0169880521 | 0,0141154772 | 0,011728637 |
| 41 | 0,0179364333 | 0,0149403391 | 0,0124447112 |
| 42 | 0,0189129834 | 0,0157917752 | 0,0131856597 |
| 43 | 0,0199178384 | 0,0166699782 | 0,0139517235 |
| 44 | 0,0209511319 | 0,0175751379 | 0,01474314 |
| 45 | 0,0220129949 | 0,0185074407 | 0,0155601436 |
| 46 | 0,0231035562 | 0,0194670703 | 0,0164029652 |
| 47 | 0,0242229421 | 0,0204542075 | 0,0172718328 |
| 48 | 0,0253712769 | 0,0214690304 | 0,0181669716 |
| 49 | 0,0265486828 | 0,0225117143 | 0,0190886036 |
| 50 | 0,02775528 | 0,0235824322 | 0,0200369483 |
| 51 | 0,0289911865 | 0,0246813545 | 0,0210122224 |
| 52 | 0,0302565189 | 0,0258086492 | 0,0220146402 |
| 53 | 0,0315513914 | 0,0269644822 | 0,0230444132 |
| 54 | 0,0328759169 | 0,028149017 | 0,0241017507 |
| 55 | 0,0342302066 | 0,0293624149 | 0,0251868596 |
| 56 | 0,0356143697 | 0,0306048353 | 0,0262999445 |
| 57 | 0,0370285142 | 0,0318764356 | 0,0274412077 |
| 58 | 0,0384727463 | 0,0331773711 | 0,0286108494 |
| 59 | 0,039947171 | 0,0345077952 | 0,0298090678 |
| 60 | 0,0414518916 | 0,0358678596 | 0,0310360589 |
| 61 | 0,0429870102 | 0,0372577141 | 0,0322920169 |
| 62 | 0,0445526273 | 0,0386775068 | 0,0335771338 |
| 63 | 0,0461488424 | 0,0401273841 | 0,0348916 |
| 64 | 0,0477757536 | 0,0416074907 | 0,036235604 |
| 65 | 0,0494334576 | 0,0431179699 | 0,0376093323 |
| 66 | 0,0511220501 | 0,0446589632 | 0,0390129699 |
| 67 | 0,0528416255 | 0,0462306108 | 0,0404467 |
| 68 | 0,0545922773 | 0,0478330511 | 0,0419107041 |
| 69 | 0,0563740976 | 0,0494664215 | 0,0434051623 |
| 70 | 0,0581871775 | 0,0511308576 | 0,0449302529 |
| 71 | 0,0600316071 | 0,0528264938 | 0,0464861526 |
| 72 | 0,0619074756 | 0,0545534633 | 0,0480730369 |
| 73 | 0,0638148709 | 0,0563118977 | 0,0496910795 |
| 74 | 0,0657538803 | 0,0581019275 | 0,0513404528 |
| 75 | 0,0677245897 | 0,059923682 | 0,0530213278 |
| 76 | 0,0697270844 | 0,0617772892 | 0,054733874 |
| 77 | 0,0717614488 | 0,0636628758 | 0,0564782599 |
| 78 | 0,0738277663 | 0,0655805676 | 0,0582546522 |
| 79 | 0,0759261195 | 0,0675304891 | 0,0600632165 |
| 80 | 0,07805659 | 0,0695127636 | 0,0619041174 |
Little theory about 3d crosstalk
When you are viewing picture for your left eye, the patch stripe consists of two pictures, the left eye patch and the black portion of the right side picture.The black is not completely black and it depends of televisions contrast ratio. In calculations we normalize the luminance that complete black is 0 and full white is 1. There is no complete black ( unless you have OLED) so in our calculations black is the contrast ratio CR. If my tv has CR 1:3000 then luminance of black will be 1/3000.
We'll call 3d crosstalk contrast as 3dCR.
The patch luminance of "right" picture will be ( CR + power(patch,gamma) *(1-CR)) because the patch number has to be transformed to linear values through gamma power function and then it has to fit between values CR and 1. The luminance of "wrong" picture will be black multilpied by 3dCR.
Then the luminance of the patch you selected is:
( CR + power(patch,gamma) *(1-CR)) + CR*3dCR
The white stripe ( the "wrong" picture ) consists of black of "right" picture and white of "wrong" picture multiplied by 3dCR.
Then the white stripe luminance is: CR + 1 * 3dCR
Because you selected the patch that equals the white stripe:
( CR + power(patch,gamma) *(1-CR)) + CR*3dCR = CR + 1 * 3dCR
I'll then solve 3dCR:
( CR + power(patch,gamma) *(1-CR)) + CR*3dCR = CR + 1 * 3dCR
CR + power(patch,gamma) *(1-CR) + CR*3dCR = CR + 1 * 3dCR
power(patch,gamma) *(1-CR) + CR*3dCR = 1 * 3dCR
power(patch,gamma) *(1-CR) = 1 * 3dCR - CR*3dCR
power(patch,gamma) *(1-CR) = 3dCR * (1 - CR)
power(patch,gamma) = 3dCR
The equation seemed difficult at first but in the end only the patch luminance is needed for 3d contrast ratio!
I've precalculated 3d contrast ratio ( = 1/3dCR) values for different gamma values:
| Patch | 2,2 | 2,3 | 2,4 |
| 0 | infinite | infinite | infinite |
| 1 | 196 965 | 342 801 | 596 618 |
| 2 | 42 867 | 69 610 | 113 038 |
| 3 | 17 568 | 27 395 | 42 717 |
| 4 | 9 329 | 14 135 | 21 417 |
| 5 | 5 710 | 8 461 | 12 536 |
| 6 | 3 823 | 5 563 | 8 093 |
| 7 | 2 724 | 3 902 | 5 591 |
| 8 | 2 030 | 2 870 | 4 058 |
| 9 | 1 567 | 2 189 | 3 059 |
| 10 | 1 243 | 1 718 | 2 375 |
| 11 | 1 008 | 1 380 | 1 890 |
| 12 | 832 | 1 130 | 1 533 |
| 13 | 698 | 940 | 1 265 |
| 14 | 593 | 792 | 1 059 |
| 15 | 509 | 676 | 898 |
| 16 | 442 | 583 | 769 |
| 17 | 387 | 507 | 665 |
| 18 | 341 | 445 | 579 |
| 19 | 303 | 393 | 509 |
| 20 | 270 | 349 | 450 |
| 21 | 243 | 312 | 400 |
| 22 | 219 | 280 | 358 |
| 23 | 199 | 253 | 322 |
| 24 | 181 | 229 | 291 |
| 25 | 166 | 209 | 263 |
| 26 | 152 | 191 | 240 |
| 27 | 140 | 175 | 219 |
| 28 | 129 | 161 | 201 |
| 29 | 119 | 148 | 184 |
| 30 | 111 | 137 | 170 |
| 31 | 103 | 127 | 157 |
| 32 | 96 | 118 | 146 |
| 33 | 90 | 110 | 135 |
| 34 | 84 | 103 | 126 |
| 35 | 79 | 96 | 117 |
| 36 | 74 | 90 | 110 |
| 37 | 70 | 85 | 103 |
| 38 | 66 | 80 | 96 |
| 39 | 62 | 75 | 91 |
| 40 | 59 | 71 | 85 |
| 41 | 56 | 67 | 80 |
| 42 | 53 | 63 | 76 |
| 43 | 50 | 60 | 72 |
| 44 | 48 | 57 | 68 |
| 45 | 45 | 54 | 64 |
| 46 | 43 | 51 | 61 |
| 47 | 41 | 49 | 58 |
| 48 | 39 | 47 | 55 |
| 49 | 38 | 44 | 52 |
| 50 | 36 | 42 | 50 |
| 51 | 34 | 41 | 48 |
| 52 | 33 | 39 | 45 |
| 53 | 32 | 37 | 43 |
| 54 | 30 | 36 | 41 |
| 55 | 29 | 34 | 40 |
| 56 | 28 | 33 | 38 |
| 57 | 27 | 31 | 36 |
| 58 | 26 | 30 | 35 |
| 59 | 25 | 29 | 34 |
| 60 | 24 | 28 | 32 |
| 61 | 23 | 27 | 31 |
| 62 | 22 | 26 | 30 |
| 63 | 22 | 25 | 29 |
| 64 | 21 | 24 | 28 |
| 65 | 20 | 23 | 27 |
| 66 | 20 | 22 | 26 |
| 67 | 19 | 22 | 25 |
| 68 | 18 | 21 | 24 |
| 69 | 18 | 20 | 23 |
| 70 | 17 | 20 | 22 |
| 71 | 17 | 19 | 22 |
| 72 | 16 | 18 | 21 |
| 73 | 16 | 18 | 20 |
| 74 | 15 | 17 | 19 |
| 75 | 15 | 17 | 19 |
| 76 | 14 | 16 | 18 |
| 77 | 14 | 16 | 18 |
| 78 | 14 | 15 | 17 |
| 79 | 13 | 15 | 17 |
| 80 | 13 | 14 | 16 |
So if I have selected patch number 37 for blue color and my gamma is 2.4, the 3d contrast ratio for blue is 1:103 which means less that 1% of blue crosstalk. The table also shows why knowing your gamma setting is important.
You can calculate your white 3d contrast with this formula:
The white contrast = (
( Red contrast * 0,212655 ) +
( Green contrast * 0,715158 ) +
( Blue contrast * 0,072187 )
)
( Red contrast * 0,212655 ) +
( Green contrast * 0,715158 ) +
( Blue contrast * 0,072187 )
)
The test pictures with 60 patches:
And the white ones just for curiosity:
And the additional test pictures with 80 patches:
















Comments
Post a Comment